What evidence-based mathematics practices can teachers employ?
Page 8: Effective Classroom Practices
A number of other classroom practices are supported by moderate levels of evidence, even if they have not yet met the requirements to be considered evidence-based. Implementing these kinds of effective practices in conjunction with an EBP is yet another way teachers can improve their students’ mathematical understanding. Among these effective classroom practices is:
- Encouraging student discussion
- Presenting and comparing multiple solutions
- Assessing student understanding
Research Shows
- Students’ mathematics achievement improved significantly when student discussion was an integral part of instruction.
(Ing, et al., 2015; Huinker, 1992) - When teachers presented multiple solution strategies for solving the same problem, students demonstrated significant increases in procedural flexibility, conceptual knowledge, and procedural knowledge.
(Durkin, Star, & Rittle-Johnson, 2017; Jitendra et al., 2011) - More than 30 years of research indicates that curriculum-based measurement (CBM), provides stable, accurate student screening and progress monitoring data in mathematics.
(Lembke & Stecker, 2007; Tindal, 2013) - Teachers have successfully used error analysis to identify the problem-solving difficulties and conceptual errors made by their students.
(Kingsdorf & Krawec, 2014)
For Your Information
When implementing the effective practices listed above, teachers will expect students to explore new concepts, attempt challenging problems, discuss their thought processes, or be open to corrective feedback. However, many students might not feel comfortable engaging in these activities, therefore, teachers need to establish a supportive and safe classroom environment. Within this type of environment, teachers can stress that making mistakes is not only acceptable but also valuable because doing so creates opportunities to identify and address faulty thinking or misconceptions.
Encouraging Student Discussion
How does this practice align?
CCSSM: Standards for Mathematical Practice
- MP3: Construct viable arguments and critique the reasoning of others
Student discussion or discourse is a practice that encourages students to express their mathematical reasoning. It allows them to become aware both of their own problem-solving processes as well as those of others, and to refine their conceptual understanding. Additionally, student discussion allows the teacher to assess student understanding. This practice could be implemented during whole-group discussion or during small-group activities. To implement this practice, teachers should:
- Establish discussion procedures (e.g., students justify answers by explaining their reasoning, students ask other students for clarification).
- Establish behavioral expectations (e.g., respect others while they are talking).
- Provide supports for students with disabilities (e.g., word wall of mathematics vocabulary, opportunities to discuss thinking with a partner before sharing in a whole-group).
- Create a list of prompts to encourage discussion among the students (e.g., “What do you think about Shay’s explanation?” “Can you add to Ramsee’s explanation?”).
- Provide sufficient wait time so that students have an opportunity to formulate a response.
The video below depicts a teacher encouraging his students, during whole-group instruction, to discuss their thoughts and ideas about a series of problems they have solved regarding a V-pattern. As you watch, note that the students have difficulty articulating how they arrived at their answer, but the teacher continues to prompt and guide the discussion (time: 3:07).
Transcript: Patterns
Teacher: Does anybody see any pattern that’s happening here? What seems to be happening? Oh, everybody seems to be seeing something. Ayesha?
Ayesha: Two is adding to every one.
Teacher: Two is adding to every one? What do you mean by that?
Ayesha: One plus two is three.
Teacher: So how does it go to the second one?
Ayesha: Two times two is four, plus one.
Teacher: And where are you getting that plus one from?
Ayesha: The middle.
Teacher: What do you mean? Can you show us? What do you mean by the middle? Josh, can you help her out?
Josh: The two on the side. The two on the one side, two on the other side, that’s four, plus the one on the bottom.
Teacher: Does anybody see another pattern?
Sulanette: I see that they’re all odd numbers.
Teacher: Why do you think they’re all odd numbers?
Sulanette: Because it starts off with the V1, right? It shows 3. Well, 3 is an odd number. If it was an even number, I don’t know where it should go in the V pattern, so they’re all odd numbers.
Teacher: I think Sulanette was saying something pretty interesting. She was saying that she didn’t know if it could be an even number. Can there be an even number? Can I say if there were 84 birds, what V pattern would that be? Could there be 84 birds?
Ashley: I think that it can never be an even number because as in the V pattern of number 1, that is actually a complete V, and an even number couldn’t make that.
Teacher: Why not?
Ashley: Because the V is made up of three birds.
Teacher: Okay, the V is made up of three birds, yeah?
Ashley: And if you keep going and adding two, it’s made up of all odd number of birds, the V patterns.
Teacher: So here’s the first one right here. And then you say that two more birds come in like that, and so it’s going to be odd. Why?
Ashley: It’s going to be odd because if you add two more birds to three, that’ll make five, and five’s an odd number.
Teacher: Can someone tell me the number of geese that would be in the V number 10, the 10th pattern along?
Oscar: I got 21.
Teacher: And how did you find that information?
Oscar: I multiplied 10 by 2
Teacher: Why did you do that?
Oscar: Because I was thinking that every time the two extra birds come, they come and then they have a group. Then I multiplied it by 2 and it comes out to 20, and then I added a 1, the one in the middle, then it came out to 21.
Jenny: What they are saying is that there are two geese in a pair and there are 10 pairs, so he would try and multiply it by 2 times 10 and then add the leader would be 21.
Credits
This video is part of the Modeling Middle School Mathematics (MMM) project. If you want to order the DVD MMM series, contact [email protected].
Presenting and Comparing Multiple Solution Strategies
How does this practice align?
According to CCSSM, comparing multiple solution strategies allows children to gain an understanding of the relationship between:
- Addition and subtraction
- Multiplication and division
Teaching multiple ways to solve a problem helps students to develop flexibility (i.e., understanding that a problem may be solved accurately using different procedures and being able to use efficient procedures) and might support conceptual understanding of the procedure. To do this, teachers should:
- Demonstrate how to solve a mathematics problem using multiple strategies.
- Present the strategies side-by-side.
- Guide students through a process of comparing multiple strategies to solve a problem.
- Use common labels to draw attention to similarities.
- Prompt for specific comparisons tailored to your learning goals.
- Be sure that students, not just the teacher, are comparing and explaining.
- Include a summary of the main idea from the comparison, highlighting key points.
- Reinforce the concept of being able to solve a problem using multiple strategies.
- Encourage students to solve problems using a strategy of their choice.
- Ask students to share their strategy with peers in a small-group or whole-group setting. By doing this, students have opportunities to see how other students solved the problem, which increases their exposure to multiple solution strategies.
Note: This does not mean that each student must solve every problem using multiple strategies, a common misinterpretation of the CCSSM requirements. Rather, students exposed to multiple strategies have a greater possibility of finding at least one problem-solving approach that they can understand and apply.
Watch the video below for an example of how a teacher can present and compare multiple strategies for solving a two-digit addition problem (time: 4:31).
Transcript: Comparing Multiple Solutions
Teacher: Today during math class, we are going to be adding two-digit numbers. And what we’re going to do today is we’re going to compare multiple strategies that you can use to solve problems, and in doing so you can compare the strategies for similarities and differences and really think about when you might use one strategy over the other.
So we’re going to look at two strategies: breaking numbers apart, and we’re also going to look at the vertical addition algorithm. And to start, I’m going to be over here with breaking numbers apart. Our problem is 34+28. So to break the numbers apart, you want to break the numbers apart into the tens and the ones. So 34 breaks apart into 30 and 4. And then the number 28 breaks apart into the number 20 and 8.
The next step is to think about how you can add the ones together and how you can add the tens together. So I’m going to draw lines to help me connect the numbers. So 4+8=12. And then I need to add the tens together. So I have 20, and I’m adding that to 30, and that gives me a sum of 50. So once we’re here, the last step is easy. All you do is add the numbers together, and we know that 50+12=62. So 34+28=62 over here.
Next, what I’m going to show you is the vertical addition algorithm. So a different strategy, but we’re still adding the same numbers, 34+28. And if you notice over here, I’ve stacked the numbers on top of each other so that they’re in vertical columns. So to start, I’m going to start in the ones column. I’m going to add 4+8. 4+8=12. And when I’m solving with the algorithm, I need to think, “Only one digit can go here under my ones column, and 12 is a two-digit number.” So I need to regroup, and regrouping means I’m thinking about how many groups of tens I have and how many ones I have.
So with the number 12, I know I have 2 ones, and I know I have one group of 10, so I’m going to write a 1 above my tens column. And now that we’ve regrouped and finished the ones column, I can then move on to add in the tens column. And here I have the numbers 3+2, but I can’t forget about that one extra group of 10 that I regrouped. So 3+2=5, and if we have one more that equals 6. So here we solved 34+28=62, and if you notice 62 is the same as 62. So both of our strategies gave us the same answer.
Now, I want you to think what else is similar or the same about these two strategies besides just the same answer. Yes, Emma?
Emma: You added the ones first and then the tens.
Teacher: Oh, that’s such great thinking, Emma! We did, for both of the strategies, I targeted thinking about my ones column or the numbers in the ones first, and then I moved on to my tens. And that’s really important when you’re thinking about addition is to always start in the ones. Now even though we started in the ones for both of them, I think you noticed that we regrouped for the algorithm, but we did not regroup when we were breaking numbers apart. Raise your hand if you can tell me why we had to regroup when using the algorithm.
So now that we’ve looked at both strategies, and we’ve had our discussion about similarities and differences, it’s really important to think about in math that, while there are multiple ways to solve a problem, thinking about what is the best way to solve it in certain situations. So, again, if you’re doing math in your head you might want to use a breaking apart numbers strategy, and if you have a pencil and a piece of paper you might want to use the algorithm if that’s going to be more efficient for you.
So now that I’ve shown you one example, you are going to do this problem next, and when you’re done you are going to choose…well, you’re going to solve it using one of the strategies, and when you’re done you’re going to work with a partner and you’re going to discuss the similarities and differences between how you solved this problem. I’ll be walking around to answer questions or to provide help as needed.
Assessing Student Understanding
How does this practice align?
High-Leverage Practice (HLP)
- HLP6: Use student assessment data, analyze instructional practices, and make necessary adjustments that improve student outcomes.
As we previously explained, assessing student understanding allows teachers to determine whether students have learned the mathematical procedures or concepts covered in class. Teachers can use different types of assessment data, including formative assessment and error analysis, to make instructional decisions (e.g., identifying what they need to revisit or reteach).
Formative Assessment
Formative assessment is the ongoing evaluation of student learning as a means of providing continual feedback about performance to both learners and instructors. By using formative assessment teachers can determine what students have mastered and what concepts they are struggling with. Teachers can use both informal and formal formative assessments. Informal assessments include exit tickets, quizzes, and class work samples. Formal formative assessments include curriculum-based measurement (CBM), sometimes referred to as general outcome measures (GOM), which is a type of progress monitoring.
exit ticket
A tool for assessing student understanding of a topic or skill introduced in class; sometimes referred to as an exit card. To assess the students’ understanding of the day’s lesson, teachers hand out blank index cards and ask their students to do such things as:
- Answer a specific question about the lesson
- Demonstrate a skill (adding two two-digit numbers)
- List three things they learned
- Ask a question about something they don’t understand about the topic
- Draw a picture of an item and label its parts
- Explain a concept
- Write down one thing they would like to know more about
The students write their names and responses on the cards and turn them in to the teacher. For older students, these cards are often turned in as students exit the class.
Example
Lesson: fractions
The teacher writes on the board and reads aloud: “If Sue gets $4.00 this week for her allowance and she spends one fourth of it at the store, how much did she spend? Show how you solved the problem.”
By reviewing these two exit tickets, the teacher realizes that Sara needs to use a graphic representation to solve the problem, whereas Nathan is able to use a mathematical representation to solve it.
progress monitoring
A type of formative assessment in which student learning is evaluated on a regular basis in order to provide useful feedback about performance to both learners and instructors. The resulting data can be graphed to observe change over time. Sometimes referred to as curriculum-based measurement (CBM) or general outcome measurement (GOM).
For more information on CBM for mathematics, view the following IRIS Module:
Error Analysis
Error analysis is the process by which instructors identify the types of errors made by students when working mathematical problems. It allows teachers to assess student understanding, or misunderstanding, and to identify and analyze the student’s error patterns—errors a student repeatedly makes when solving a mathematical problem. The teacher can use the information from the error analysis to target instruction to help the student understand the correct procedure for solving the problem. If the reasons for the student’s incorrect answers are not apparent, the teacher can ask the student to describe the procedure she used to solve the problem, as is illustrated in the box below.
Example: Error Analysis
Student Solutions:
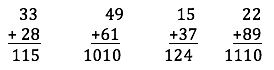
Student Explanation:
For the first problem, I added 8+3 and got 11, so I wrote 11 down. Then I added 3+2 and got 5. I wrote the 5 after the 11. So I got 115. I did the same thing for the other problems.
Diane Bryant discusses the instructional implications for using formative feedback and error analysis (time: 2:11).
Diane Pedrotty Bryant, PhD
Project Director, Mathematics Institute for Learning Disabilities and Difficulties
University of Texas at Austin
Transcript: Diane Pedrotty Bryant, PhD
Formative assessment and error analysis are critical aspects of instruction and help teachers understand if students are benefiting from the math interventions that they’re using. Formative ongoing assessment, really, is important so that teachers are aware of the skills that have been taught that students are still not understanding. If students are stuck on a particular mathematical concept or skill and don’t learn it to mastery and don’t understand how to generalize mathematical ideas, they will continue to struggle in mathematics as the curriculum becomes more advanced in later academic years. In terms of error analysis, it’s important for teachers to understand where the breakdowns are occurring, whether it’s a mathematical procedure, a set of steps, how solutions are arrived at. Error analysis can be really informative in terms of the mistakes that students are making or the misconceptions that students have. It’s important for teachers to work with students individually, to have students articulate how they went about solving problems, because that is such an important part of error analysis, because then you could potentially tap the thinking process that students are using and really get at some of those misconceptions that students learn over the years. I think pairing formative assessment with error analysis can really help teachers figure out where the faulty thinking is and where the misconceptions might be, which can really help to inform instructional decision making about the next steps for math instruction.
To learn more about error analysis, visit the following IRIS Case Study Unit:
