What evidence-based mathematics practices can teachers employ?
Page 7: Metacognitive Strategies
As you have now learned, students who struggle with mathematics tend to be poor problem solvers. They approach every mathematics problems using only a small number of strategies, and even these strategies they apply inconsistently. Teachers can begin to address these issues by teaching the students cognitive strategies (e.g., schema-based instruction, mnemonics) that help students focus their attention on relevant information, identify a given problem’s structure, and solve the problem.
However, teaching students cognitive strategies alone is not enough to ensure that those strategies will be implemented correctly or independently. This is especially the case for students with mathematics difficulties and disabilities, who tend to implement the same strategy for every problem, implement strategies without considering the problem type, or fail to use a strategy at all. If students are to be more successful, teachers should pair instruction on cognitive strategies with that of metacognitive strategies—strategies that enable students to become aware of how they think when solving mathematics problems. This combined strategy instruction teaches students how to consider the appropriateness of the problem-solving approach, make sure that all procedural steps are implemented, and check for accuracy or to confirm that their answers makes sense. More specifically, metacognitive strategies help students learn to:
How does this practice align?
High-Leverage Practice (HLP)
- HLP14: Teach cognitive and metacognitive strategies to support learning and independence
CCSSM: Standards for Mathematical Practice
- MP1: Make sense of problems and persevere in solving them.
- Plan — Students decide how to approach the mathematical problem, first determining what the problem is asking and then selecting and implementing an appropriate strategy to solve it.
- Monitor — As students solve a mathematical problem, they check to see whether their problem-solving approach is working. After completing the problem, they consider whether the answer makes sense.
- Modify — If, as they work to solve a mathematical problem, students determine that their problem-solving approach is not working or that their answer is incorrect, they can adjust their approach.
Research Shows
- When paired with cognitive strategies, metacognitive strategies have been shown to increase the understanding and ability of students with mathematics learning difficulties and disabilities to solve mathematics problems.
(Pfannenstiel, Bryant, Bryant, & Porterfield, 2015) - Middle school students who received cognitive and metacognitive strategy instruction outperformed peers who received typical math instruction.
(Montague, Enders, & Dietz, 2011; Pfannenstiel, Bryant, Bryant, & Porterfield, 2015)
Types of Metacognitive Strategies
Metacognitive strategies that help students plan, monitor, and modify their mathematical problem-solving include self-instruction and self-monitoring. Not only are these strategies relatively easy for students to implement, but they also help students to become better independent problem solvers.
| Metacognitive Strategy | Definition | Examples |
|---|---|---|
| Self-instruction | Talking one’s self through a task or activity (also known as self-talk) |
|
| Self-monitoring | Checking one’s performance; often involves a checklist |
|
Teaching Metacognitive Strategies
Teachers should use explicit instruction to help students understand how to use self-instruction and self-monitoring during the problem-solving process. To do this, teachers can:
- Provide students with a list of questions or prompts to ask themselves while they are engaged in the problem-solving process.
- Example questions: What information is relevant? Have I solved a problem like this before?
- Example prompts: Identify the relevant information. Use a visual to solve the problem.
- Model working through a problem using “think alouds,” during which the teacher verbalizes her thoughts as she demonstrates using self-instruction and self-monitoring throughout the problem-solving process.
- Provide sufficient opportunities for students to practice these metacognitive strategies with corrective feedback.
- Encourage students to use these strategies independently, once they have achieved mastery.
Examples of Students Using Metacognitive Strategies
The videos below illustrate students using metacognitive strategies to solve mathematics problems. In the first video, in addition to self-instruction, an elementary student uses an age-appropriate self-monitoring checklist that includes visual cues for each step. Note that the student was explicitly taught how to use this checklist before using it to solve problems independently. In the second video, a high-school student uses self-instruction and self-monitoring to solve a word problem.
Elementary School Example (time: 1:49)
Transcript: Metacognative Strategies: Elementary School
Narrator: In this video, an elementary student uses metacognitive strategies while solving an addition problem. More specifically, he uses self-instruction and a self-monitoring checklist to guide himself through the problem-solving process. By doing so, he actively plans and monitors his work.
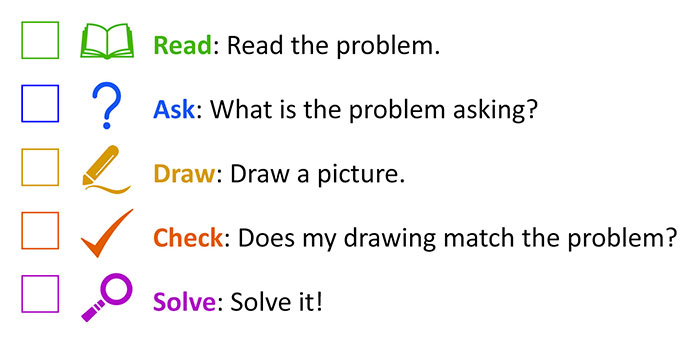
Student: I can’t figure out what 3 + 5 is. What is it? Well, let me look at my checklist. First, it says, “read the problem.” The problem says 3 + 5, so I’ve checked that. Now what is…now it says…my checklist says, “What is the problem asking?” It’s asking me to add 3 + 5.
Now, to draw a picture. One, two, three. One, two, three, four, five. Now it says, “Does my drawing match the problem?” Up here it says 3 + 5, so down here it says one, two, three, one, two, three, four, five. Now I have to solve it. So one, two, three, four, five, six, seven, eight. The answer to 3 + 5 is 8.
High School Example (time: 2:54)
Transcript: Metacognative Strategies: High School
Narrator: In this video, a high school student uses metacognitive strategies while solving a word problem. By using self-instruction and self-monitoring, she actively plans and monitors her work.
Student: First, I’m going to read the problem. “Mr. Smith, the principal, is standing on top of the high school. He is looking at a tree in the courtyard that is 30 feet away from the school. The angle from Mr. Smith’s feet to the base of the tree is 43 degrees. Using this information, determine the height of the high school.”
So what am I missing? The problem says that the angle from Mr. Smith’s feet to the base of the tree is 43 degrees. I’ve noticed that, if you connect this point to this point, we have a right triangle. So, while this angle is 43 degrees, this angle right here is a right angle that’s 90 degrees.
There’s a trick that I’m going to use that’s called SOHCAHTOA that you can use to find the sides and angles in a right triangle. The opposite side to 43 degrees is 30 feet, right here. So what do I need to find? I need to find the adjacent side. I’ll label it with an “A.” I look at SOHCAHTOA, and I know that I need to find the tangent, because tangent equals opposite over adjacent.
Now all I have to do is plug in the information that I have in order to find “A.” Tangent of 43 degrees, the angle, equals 30—that’s the opposite side—over
“A.” And I find that 30 over 0.93 equals 32.25. So the height of this building is 32.25 feet.
Now that I’ve solved the problem, I ask does my answer make sense? Given the information from the problem, and with what I know about most buildings, 32 feet seems like a reasonable answer.
Diane Bryant discusses the importance of teaching students cognitive and metacognitive strategies and how they benefit students (time: 2:22).
Diane Pedrotty Bryant, PhD
Project Director, Mathematics Institute for Learning Disabilities and Difficulties
University of Texas at Austin
Transcript: Diane Pedrotty Bryant, PhD
It really is important to pair metacognitive strategies with cognitive strategies. Metacognitive strategies simply refers to thinking about thinking. It’s beneficial and certainly validated in research that they have a series of cognitive steps to employ to solve problems, whatever the problem might be. The metacognitive strategies help students think about what steps that they’re supposed to be using—that’s the self-instruction—and then pausing to check about whether they are indeed using those various cognitive strategies steps, which really refers to the self-monitoring. For students with mathematics learning disabilities, we want them to become independent learners and to use strategies for solving various problems and to be able to pause and ask themselves questions about how they’re proceeding and back up and check on a particular step. Through the use of cognitive strategies paired with metacognitive strategies, the goal is to empower them to be more independent learners, and that’s definitely something we strive for when we teach students with learning disabilities. I think that there is difficulty in students learning how to implement metacognitive strategies independently, because they may not know how to approach the learning task. They may not be aware of their own ability to self-monitor, to self-instruct, to use self-talk, self-verbalizations for tackling tasks. Usually students with mathematics learning disabilities really need to be taught to use metacognitive strategies and to learn the metacognitive strategies to mastery before being able to use them independently.
For Your Information
Although teachers can provide students with a generic list of questions or prompts to guide them through the problem-solving process, some students, such as those with mathematics difficulties and disabilities, might need more individualized support to address their specific learning challenges. The teacher can identify the student’s common error patterns by conducting an error analysis—a process by which instructors identify the types of errors made by students when working mathematical problems. Using this information, teachers can develop a list of questions or prompts that students can use to address their specific needs. To begin with, many of these students might require a self-monitoring checklist, such as the one below, to guide them through the problem-solving process.
